

Surface Area and Volume of Three Dimensional Figures.Surface Area and Volume of Different Combination of Solid Figures.Conversion of Solid from One Shape to Another.Surface Area of a Combination of Solids.Concept of Surface Area, Volume, and Capacity.Areas of Sector and Segment of a Circle.Graphical Representation of Cumulative Frequency Distribution.Trigonometric Ratios of Some Special Angles.Trigonometric Ratios and Its Reciprocal.Trigonometric Ratios of Complementary Angles.Application of Pythagoras Theorem in Acute Angle and Obtuse Angle.Right-angled Triangles and Pythagoras Property.Basic Proportionality Theorem (Thales Theorem).Number of Tangents from a Point on a Circle.Concept of Circle - Centre, Radius, Diameter, Arc, Sector, Chord, Segment, Semicircle, Circumference, Interior and Exterior, Concentric Circles.Relationship Between Zeroes and Coefficients of a Polynomial.Geometrical Meaning of the Zeroes of a Polynomial.Situational Problems Based on Quadratic Equations Related to Day to Day Activities to Be Incorporated.
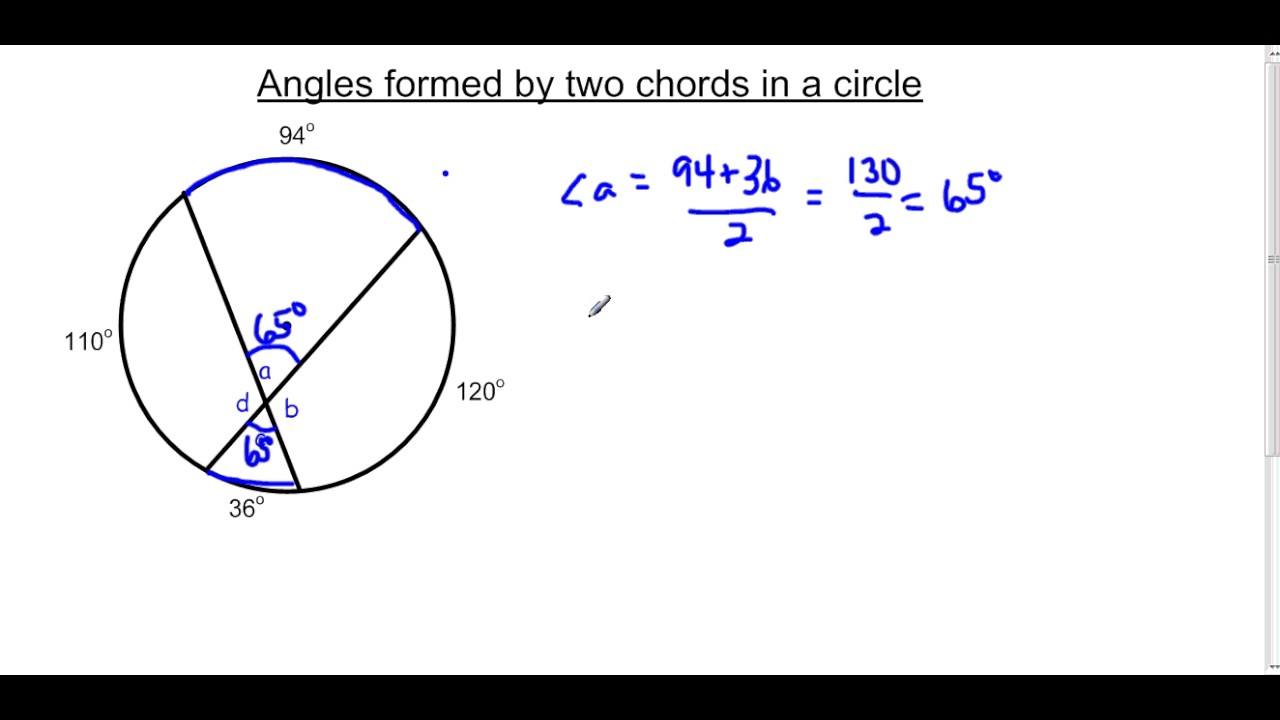

Inconsistency of Pair of Linear Equations.Consistency of Pair of Linear Equations.Equations Reducible to a Pair of Linear Equations in Two Variables.Graphical Method of Solution of a Pair of Linear Equations.Pair of Linear Equations in Two Variables.Rational Numbers and Their Decimal Expansions.Fundamental Theorem of Arithmetic Motivating Through Examples.m ∠ R = 90° (Theorem 72).įigure 6 An inscribed angle which intercepts a semicircle.Įxample 4: In Figure 7 of circle O, m 60° and m ∠1 = 25°.įigure 7 A circle with inscribed angles, central angles, and associated arcs. Theorem 72: If an inscribed angle intercepts a semicircle, then its measure is 90°.įigure 4 Finding the measure of an inscribed angle.Įxample 2: Find m ∠ A and m ∠ B in Figure 5.įigure 5 Two inscribed angles with the same measure.Įxample 3: In Figure 6, QS is a diameter. Theorem 71: If two inscribed angles of a circle intercept the same arc or arcs of equal measure, then the inscribed angles have equal measure. The following two theorems directly follow from Theorem 70. Theorem 70: The measure of an inscribed angle in a circle equals half the measure of its intercepted arc. Notice that m ∠3 is exactly half of m, and m ∠4 is half of m ∠3 and ∠4 are inscribed angles, and and are their intercepted arcs, which leads to the following theorem. Refer to Figure 3 and the example that accompanies it.įigure 3 A circle with two diameters and a (nondiameter) chord. In Figure 1, ∠ ABC is an inscribed angle and is its intercepted arc.įigure 1 An inscribed angle and its intercepted arc.įigure 2 shows examples of angles that are not inscribed angles.įigure 2 Angles that are not inscribed angles. Intercepted arc: Corresponding to an angle, this is the portion of the circle that lies in the interior of the angle together with the endpoints of the arc.Inscribed angle: In a circle, this is an angle formed by two chords with the vertex on the circle.Angles may be inscribed in the circumference of the circle or formed by intersecting chords and other lines. Summary of Coordinate Geometry FormulasĬentral angles are probably the angles most often associated with a circle, but by no means are they the only ones.Slopes: Parallel and Perpendicular Lines.Similar Triangles: Perimeters and Areas.Proportional Parts of Similar Triangles.Formulas: Perimeter, Circumference, Area.Proving that Figures Are Parallelograms.Triangle Inequalities: Sides and Angles.Special Features of Isosceles Triangles.Classifying Triangles by Sides or Angles.Lines: Intersecting, Perpendicular, Parallel.


 0 kommentar(er)
0 kommentar(er)
